|
2022年高考数学甲卷-理17<-->2022年高考数学甲卷-理19
(12分)在四棱锥P−ABCD中,PD⊥底面ABCD,CD//AB,AD=DC=CB=1,AB=2,DP=√3.
(1)证明:BD⊥PA;
(2)求PD与平面PAB所成的角的正弦值.
分析:(1)易知PD⊥BD,取AB中点E,容易证明四边形BCDE为平行四边形,再根据长度关系可得BD⊥AD,进而得证;
(2)建立空间直角坐标系,写出各点的坐标,再求出平面PAB的法向量,利用向量的夹角公式即可得解.
解答:解:(1)证明:∵PD⊥底面ABCD,BD⊂面ABCD,
∴PD⊥BD,
取AB中点E,连接DE,
∵AD=DC=CB=1,AB=2,
∴∠DAB=60∘,又∵AE=12AB=AD=1,
∴DE=1,∴DE=12AB,
∴ΔABD为直角三角形,且AB为斜边,
∴BD⊥AD,
又PD⋂AD=D,PD⊂面PAD,AD⊂面PAD,
∴BD⊥面PAD,
又PA⊂面PAD,
∴BD⊥PA;
(2)由(1)知,PD,AD,BD两两互相垂直,故建立如图所示的空间直角坐标系,
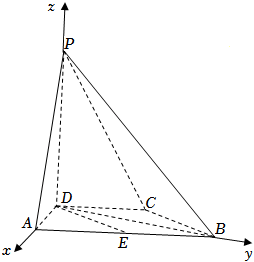
BD=√AB2−AD2=√3,
则D(0,0,0),A(1,0,0),B(0,√3,0),P(0,0,√3),
∴→PD=(0,0,−√3),→PA=(1,0,−√3),→AB=(−1,√3,0),
设平面PAB的一个法向量为→n=(x,y,z),则{→n⋅→PA=x−√3z=0→n⋅→AB=−x+√3y=0,则可取→n=(√3,1,1),
设PD与平面PAB所成的角为θ,则sinθ=|cos<→PD,→n>|=|→PD⋅→n|→PD||→n||=√55,
∴PD与平面PAB所成的角的正弦值为√55.
解答:本题考查线面垂直的判定以及利用空间向量求解二面角的正弦值,考查逻辑推理能力及运算求解能力,属于中档题.
2022年高考数学甲卷-理17<-->2022年高考数学甲卷-理19
全网搜索"2022年高考数学甲卷-理18"相关
|

