| 2023年高考数学新高考Ⅰ-18 |
|
2023-07-08 11:03:38 |
|
|
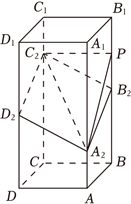
(12分)如图,在正四棱柱ABCD−A1B1C1D1中,AB=2,AA1=4.点A2,B2,C2,D2分别在棱AA1,BB1,CC1,DD1上,AA2=1,BB2=DD2=2,CC2=3.
(1)证明:B2C2//A2D2;
(2)点P在棱BB1上,当二面角P−A2C2−D2为150∘时,求B2P.
分析:(1)建系,根据坐标法及向量共线定理,即可证明;
(2)建系,根据向量法,向量夹角公式,方程思想,即可求解.
解:
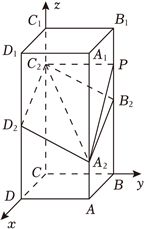
(1)证明:根据题意建系如图,则有:
B2(0,2,2),C2(0,0,3),A2(2,2,1),D2(2,0,2),
∴→B2C2=(0,−2,1),→A2D2=(0,−2,1),
∴→B2C2=→A2D2,又B2,C2,A2,D2四点不共线,
∴B2C2//A2D2;
(2)在(1)的坐标系下,可设P(0,2,t),t∈[0,4],
又由(1)知C2(0,0,3),A2(2,2,1),D2(2,0,2),
∴→C2A2=(2,2,−2),→C2P=(0,2,t−3),→A2D2=(0,−2,1),
设平面PA2C2的法向量为→m=(x,y,z),
则{→m⋅→C2A2=2x+2y−2z=0→m⋅→C2P=2y+(t−3)z=0,取→m=(t−1,3−t,2),
设平面A2C2D2的法向量为→n=(a,b,c),
则{→n⋅→C2A2=2a+2b−2c=0→n⋅→A2D2=−2b+c=0,取→n=(1,1,2),
∴根据题意可得|cos150∘|=|cos<→m,→n>|=|→m⋅→n||→m||→n|,
∴√32=6√(t−1)2+(3−t)2+4×√6,
∴t2−4t+3=0,又t∈[0,4],
∴解得t=1或t=3,
∴P为B1B2的中点或B2B的中点,
∴B2P=1.
点评:本题考查利用向量法证明线线平行,利用向量法求解二面角问题,向量共线定理及向量夹角公式的应用,方程思想,属中档题.
|
|
http://x.91apu.com//shuxue/gkt/2023/2023xgk1/2023-07-08/33636.html |

