（5分）在$\Delta ABC$中，$\angle A=90^\circ$，$AB=AC=2$，点$M$为边$AB$的中点，点$P$在边$BC$上，则$\overrightarrow{MP}\cdot \overrightarrow{CP}$的最小值为 　$-\dfrac{9}{8}$　．
分析：建立平面直角坐标系，利用数量积的坐标运算求出$\overrightarrow{MP}\cdot \overrightarrow{CP}=2x^{2}-3x$，再利用二次函数求最值即可．
解：建立平面直角坐标系如下，

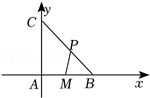
则$B(2,0)$，$C(0,2)$，$M(1,0)$，
直线$BC$的方程为$\dfrac{x}{2}+\dfrac{y}{2}=1$，即$x+y=2$，
点$P$在直线上，设$P(x,2-x)$，
$\therefore$$\overrightarrow{MP}=(x-1,2-x)$，$\overrightarrow{CP}=(x,-x)$，
$\therefore$$\overrightarrow{MP}\cdot \overrightarrow{CP}=x(x-1)-x(2-x)=2x^{2}-3x=2{(x-\dfrac{3}{4})}^{2}-\dfrac{9}{8}\geqslant -\dfrac{9}{8}$，
$\therefore$$\overrightarrow{MP}\cdot \overrightarrow{CP}$的最小值为$-\dfrac{9}{8}$．
故答案为：$-\dfrac{9}{8}$．

点评：本题考查了数量积的坐标运算，考查了二次函数求最值，属于中档题．