|
(4分)如图,已知正三棱柱ABC−A1B1C1,AC=AA1,E,F分别是棱BC,A1C1上的点.记EF与AA1所成的角为α,EF与平面ABC所成的角为β,二面角F−BC−A的平面角为γ,则( )
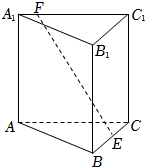
A.α⩽β⩽γ B.β⩽α⩽γ C.β⩽γ⩽α D.α⩽γ⩽β
分析:根据线线角的定义,线面角的定义,面面角的定义,转化即可求解.
解:∵正三棱柱ABC-A_{1}B_{1}C_{1}中,AC=AA_{1},
\therefore正三棱柱的所有棱长相等,设棱长为1,
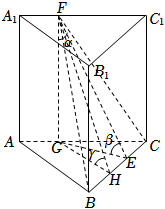
如图,过F作FG\bot AC,垂足点为G,连接GE,则A_{1}A//FG,
\therefore EF与AA_{1}所成的角为\angle EFG=\alpha,且\tan \alpha =\dfrac{GE}{FG}=GE,
又GE\in [0,1],\therefore \tan \alpha \in [0,1],
\therefore EF与平面ABC所成的角为\angle FEG=\beta,且\tan \beta =\dfrac{GF}{GE}=\dfrac{1}{GE}\in [1,+\infty ),
\therefore \tan \beta \geqslant \tan \alpha,...①,
再过G点作GH\bot BC,垂足点为H,连接HF,
又易知FG\bot底面ABC,BC\subset底面ABC,
\therefore BC\bot FG,又FG\bigcap GH=G,\therefore BC\bot平面GHF,
\therefore二面角F-BC-A的平面角为\angle GHF=\gamma,且\tan \gamma =\dfrac{GF}{GH}=\dfrac{1}{GH},又GH\in [0,1],
\therefore \tan \gamma \in [1,+\infty ),\therefore \tan \gamma \geqslant \tan \alpha,...②,
又GE\geqslant GH,\therefore \tan \beta \leqslant \tan \gamma,...③,
由①②③得\tan \alpha \leqslant \tan \beta \leqslant \tan \gamma,又\alpha,\beta,\gamma \in [0,\dfrac{\pi }{2}),y=\tan x在[0,\dfrac{\pi }{2})单调递增,
\therefore \alpha \leqslant \beta \leqslant \gamma,
故选:A.
点评:本题考查线线角的定义,线面角的定义,面面角的定义,考查了转化思想,属中档题.
|

