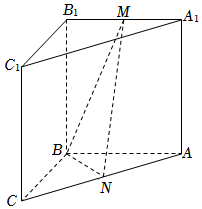
（14分）如图，在三棱柱$ABC-A_{1}B_{1}C_{1}$中，侧面$BCC_{1}B_{1}$为正方形，平面$BCC_{1}B_{1}\bot$平面$ABB_{1}A_{1}$，$AB=BC=2$，$M$，$N$分别为$A_{1}B_{1}$，$AC$的中点．
（Ⅰ）求证：$MN//$平面$BCC_{1}B_{1}$；
（Ⅱ）再从条件①、条件②这两个条件中选择一个作为已知，求直线$AB$与平面$BMN$所成角的正弦值．
条件①：$AB\bot MN$；
条件②：$BM=MN$．
注：如果选择条件①和条件②分别解答，按第一个解答计分．

分析：（1）通过证面面平证线面平行；
（2）通过证明$BC$，$BA$，$BB_{1}$两两垂直，从而建立以$B$为坐标原点，$BC$，$BA$，$BB_{1}$为坐标轴建立如图所示的空间直角坐标系，利用向量法求线面角的正弦值．
解答：解：$(I)$证明：取$AB$中点$K$，连接$NK$，$MK$，
$\because M$为$A_{1}B_{1}$的中点．$\therefore B_{1}M//BK$，且$B_{1}M=BK$，
$\therefore$四边形$BKMB_{1}$是平行四边形，故$MK//BB_{1}$，
$MK\not\subsetset$平面$BCC_{1}B_{1}$；$BB_{1}\subset$平面$BCC_{1}B_{1}$，
$\therefore MK//$平面$BCC_{1}B_{1}$，
$\because K$是$AB$中点，$N$是$AC$的点，
$\therefore NK//BC$，$\because NK\not\subsetset$平面$BCC_{1}B_{1}$；$BC\subset$平面$BCC_{1}B_{1}$，
$\therefore NK//$平面$BCC_{1}B_{1}$，又$NK\bigcap MK=K$，
$\therefore$平面$NMK//$平面$BCC_{1}B_{1}$，
又$MN\subset$平面$NMK$，$\therefore MN//$平面$BCC_{1}B_{1}$；
$(II)\because$侧面$BCC_{1}B_{1}$为正方形，平面$BCC_{1}B_{1}\bot$平面$ABB_{1}A_{1}$，平面$BCC_{1}B_{1}\bigcap$平面$ABB_{1}A_{1}=BB_{1}$，
$\therefore CB\bot$平面$ABB_{1}A_{1}$，$\therefore CB\bot AB$，又$NK//BC$，$\therefore AB\bot NK$，
若选①：$AB\bot MN$；又$MN\bigcap NK=N$，$\therefore AB\bot$平面$MNK$，
又$MK\subset$平面$MNK$，$\therefore AB\bot MK$，又$MK//BB_{1}$，
$\therefore AB\bot BB_{1}$，$\therefore BC$，$BA$，$BB_{1}$两两垂直，
若选②：$\because CB\bot$平面$ABB_{1}A_{1}$，$NK//BC$，$\therefore NK\bot$平面$ABB_{1}A_{1}$，$KM\subset$平面$ABB_{1}A_{1}$，
$\therefore MK\bot NK$，又$BM=MN$，$NK=\dfrac{1}{2}BC$，$BK=\dfrac{1}{2}AB$，
$\therefore \Delta BKM\cong \Delta NKM$，$\therefore \angle BKM=\angle NKM=90^\circ$，
$\therefore AB\bot MK$，又$MK//BB_{1}$，$\therefore AB\bot BB_{1}$，
$\therefore BC$，$BA$，$BB_{1}$两两垂直，
以$B$为坐标原点，$BC$，$BA$，$BB_{1}$为坐标轴建立如图所示的空间直角坐标系，

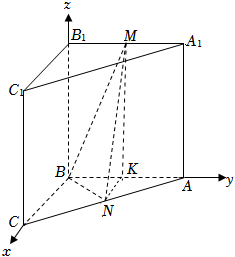
则$B(0$，0，$0)$，$N(1$，1，$0)$，$M(0$，1，$2)$，$A(0$，2，$0)$，
$\therefore$$\overrightarrow{BM}=(0$，1，$2)$，$\overrightarrow{BN}=(1$，1，$0)$，

设平面$BMN$的一个法向量为$\overrightarrow{n}=(x$，$y$，$z)$，
则$\left\{\begin{array}{l}{\overrightarrow{n}\cdot \overrightarrow{BM}=y+2z=0}\\ {\overrightarrow{n}\cdot \overrightarrow{BN}=x+y=0}\end{array}\right.$，令$z=1$，则$y=-2$，$x=2$，
$\therefore$平面$BMN$的一个法向量为$\overrightarrow{n}=(2$，$-2$，$1)$，
又$\overrightarrow{BA}=(0$，2，$0)$，
设直线$AB$与平面$BMN$所成角为$\theta$，
$\therefore \sin \theta =\vert \cos  < \overrightarrow{n}$，$\overrightarrow{BA} > \vert =\dfrac{\vert \overrightarrow{n}\cdot \overrightarrow{BA}\vert }{\vert \overrightarrow{n}\vert \cdot \vert \overrightarrow{BA}\vert }=\dfrac{4}{\sqrt{4+4+1}\times 2}=\dfrac{2}{3}$．
$\therefore$直线$AB$与平面$BMN$所成角的正弦值为$\dfrac{2}{3}$．
点评：本题考查线面平行的证明，线面角的求法，属中档题．